| Institute of Hydrogeology and Environmental Geology, Chinese Academy of Geological Sciences | Host |
| Groundwater Science and Engineering Limited | Publish |
| Citation: |
Gao Fei, Liu Feng, Wang Hua-jun. 2021. Numerical modelling of the dynamic process of oil displacement by water in sandstone reservoirs with random pore structures. Journal of Groundwater Science and Engineering, 9(3): 233-244. doi: 10.19637/j.cnki.2305-7068.2021.03.006
|
Numerical modelling of the dynamic process of oil displacement by water in sandstone reservoirs with random pore structures
-
Abstract
In order to maintain the production rate of a reservoir and improve the displacement efficiency, it is crucial to have an in-depth understanding of the process of oil displacement by water. However, with respect to the conceptualization of porous media of a reservoir, very limited efforts have been made to the pore structures inside the reservoirs. In this paper, the pore structures of a sandstone reservoir were generated by using the method of random growth algorithm. Based on the randomly generated model, a theoretical model to describe the dynamic process of oil displacement by water in the sandstone reservoir was established, and then corresponding numerical modelling was performed. The effects of the displacement velocity, the viscosity ratio of oil-water phase and the porosity of reservoirs on the displacement performance were also analyzed. Results show that due to a great difference in the viscosity between oil and water phases, the moving interface of water phase is not uniform, and the viscous fingering occurs, tending to proceed along the direction with the minimum flow resistance. There is not a linear relationship between the displacement velocity and the displacement efficiency. Too high displacement velocities do not lead to much better displacement efficiency, while a higher pressure drop is caused. Choosing a proper displacement velocity is indispensable in practical engineering. A lower oil-water viscosity ratio is more favorable to obtain high displacement efficiency. Under the present simulation conditions, when the viscosity ratio is 1.2, the displacement efficiency reaches 96.2% at a moderate Reynolds number. The porosity is not a sole factor determining the displacement performance. Even for the same porosity, the shape and length of preferential flow paths are different and randomly distributed, causing a different displacement performance. A large tortuosity tends to result in a low hydraulic conductivity and displacement efficiency.
-
-
References
[1] De Castro MS, Rodriguez MH. 2015. Interfacial waves in stratified viscous oil-water flow. Experimental Thermal and Fluid Science, 62: 85-98. doi: 10.1016/j.expthermflusci.2014.12.003 [2] Fernandez-Berdaguer EM, Savioli GB. 2009. An inverse problem arising from the displacement of oil by water in porous media. Applied Numerical Mathematics, 59(10): 2452-2466. doi: 10.1016/j.apnum.2009.04.009 [3] Flury M, Flühler H, Jury W A, et al. 1994. Susceptibility of soils to preferential flow of water: A field study. Water Resources Research, 30(7): 1945-1954. doi: 10.1029/94WR00871 [4] Gao ZJ, Liu YG. 2013. Groundwater flow driven by heat. Journal of Groundwater Science and Engineering, 1(3): 22-27. [5] Hossein R, Mahshid J, Saman A, et al. 2013. Review of sand production prediction models. Journal of Petroleum Engineering: 1-16. doi: 10.1155/2013/864981 [6] Ju Y, Gong WB, Zheng JT. 2019. Characterization of immiscible phase displacement in heterogeneous pore structures: Parallel multicomponent lattice Boltzmann simulation and experimental validation using three-dimensional printing technology. International Journal of Multiphase Flow, 114: 50-65. doi: 10.1016/j.ijmultiphaseflow.2019.02.006 [7] Koponen A, Kataja M, Timonen J. 1997. Permeability and effective porosity of porous media. Physical Review E, 56: 3319-3325. doi: 10.1103/PhysRevE.56.3319 [8] Li LL, Su C, Hao QC, et al. 2018. Numerical simulation of response of groundwater flow system in inland basin to density changes. Journal of Groundwater Science and Engineering, 6(1): 7-17. doi: 10.19637/j.cnki.2305-7068.2018.01.002 [9] Li ZF, He SL, Yang WX, et al. 2006. Physical simulation experiment of water driving by micro-model and fractal features of residual oil distribution. Journal of China University of Petroleum, 30(3): 67-71. (in Chinese) [10] Liu HH. 2015. New water-oil displacement efficiency prediction method. Open Petroleum Engineering Journal, 7(1): 88-91. doi: 10.2174/1874834101407010088 [11] Liu YZ, Sun L, Pan Y, et al. 2012. Experimental study on microscopic water/oil displacement percolation mechanism of fractured reservoir. Reservoir Evaluation and Development, 2(5): 28-31. (in Chinese) [12] Liu ZP, Wu LG, Wei CP. 2020. Physical experiments and numerical simulations of viscosity reducer flooding for ordinary heavy oil. Journal of Petroleum Science and Engineering, 192: 107194. doi: 10.1016/j.petrol.2020.107194 [13] Lu C, Li L, Liu YG, Wang GL. 2014. Capillary pressure and relative permeability model uncertainties in simulations of geological CO2 sequestration. Journal of Groundwater Science and Engineering, 2(2): 1-17. [14] Meiburg E, Homsy G M. 1988. Nonlinear unstable viscous fingers in Hele–Shaw flows. II. Numerical simulation. Physics of Fluids, 31(3): 429-439. doi: 10.1063/1.866824 [15] Mirchi A, Hadian S, Madani K, et al. 2012. World energy balance outlook and OPEC production capacity: Implications for global oil security. Energies, 5(8): 2626-2651. doi: 10.3390/en5082626 [16] Oliveira CL, Andrade JS, Herrmann HJ. 2011. Oil displacement through a porous medium with a temperature gradient. Physical Review E Statistical Nonlinear and Soft Matter Physics, 83(2): 648-670. doi: 10.1103/physreve.83.066307 [17] Rebold JH. 1962. Evaluation of water-oil displacement efficiency using subsurface logs. Journal of Petroleum Technology, 14(1): 17-21. doi: 10.2118/84-PA [18] Rivas-Gomez S, Gonzalez-Guevara JA, Cruz-Hernandez J, et al. 2001. Numerical simulation of oil displacement by water in a vuggy fractured porous medium. Proceedings of SPE Reservoir Simulation Symposium, Houston, Texas. [19] Sun W, Tang GQ. 2006. Visual study of water injection in low permeable sandstone. Journal of Canadian Petroleum Technology, 45(11): 21-26. [20] Takeshi Tsuji, Fei Jiang, Kenneth T. 2016. Characterization of immiscible fluid displacement processes with various capillary numbers and viscosity ratios in 3D natural sandstone. Advances in Water Resources, 95: 3-15. doi: 10.1016/j.advwatres.2016.03.005 [21] Wang M, Pan N. 2008. Predictions of effective physical properties of complex multiphase materials. Materials Science and Engineering, 63(1): 1-30. doi: 10.1016/j.mser.2008.07.001 [22] Wang M, Pan N. 2009. Elastic property of multiphase composites with random microstructures. Journal of Computational Physics, 228: 5978-5988. doi: 10.1016/j.jcp.2009.05.007 [23] Wang SL, Yu CL, Sang GQ, et al. 2020. An oil-water two-phase reservoir numerical simulation coupled with dynamic capillary force based on the full-implicit method. Computers and Mathematics with Applications, 79(9): 2527-2549. doi: 10.1016/j.camwa.2019.11.013 [24] Wei JG, Li AJ, Chen YD. 2013. Oil displacement efficiency and performance evaluation of composite ion profile control agents prepared with oilfield sewage. Advances in Petroleum Exploration and Development, 5(2): 52-57. [25] Xu CF, Liu HX, Qian GB, et al. 2011. Microcosmic mechanisms of water-oil displacement in conglomerate reservoirs in Karamay Oilfield, NW China. Petroleum Exploration and Development, 38(6): 725-732. doi: 10.1016/S1876-3804(12)60006-8 [26] Yang B, Feng LF, Wang S, et al. 2016. A numerical prediction model for hydraulic conductivity of sandy aquifers based on randomly generated pore structures. E. J. Geotechnical Engineering, 21(2): 677-690. [27] Yang YQ, Cheng LY, Sha O, et al. 2013. Methods of determining oil displacement efficiency of oil displacement agent. Oilfield Chemistry, 30(2): 290-294. (in Chinese) [28] Zhang LH, Tong J, Xiong Y. 2017. Effect of temperature on the oil-water relative permeability for sandstone reservoirs. International Journal of Heat and Mass Transfer, 105: 535-548. doi: 10.1016/j.ijheatmasstransfer.2016.10.029 [29] Zhang RX, Gao YY, Li JM. 1995. Effects of displacement conditions on the water-oil displacement efficiency in glutenite reservoirs. Henan Petroleum, 13(4): 32-37. (in Chinese) [30] Zhao Y, Qu ZH, Liu Z. 2002. Experimental study on water/oil displacement mechanisms in fractured reservoir by real sandstone micro-models. Petroleum Exploration & Development, 29(1): 116-119. (in Chinese) -
Access History
Figures(12)
Tables(3)
Export File
Citation
Gao Fei, Liu Feng, Wang Hua-jun. 2021. Numerical modelling of the dynamic process of oil displacement by water in sandstone reservoirs with random pore structures. Journal of Groundwater Science and Engineering, 9(3): 233-244. doi: 10.19637/j.cnki.2305-7068.2021.03.006
Format
Content
DownLoad:
-
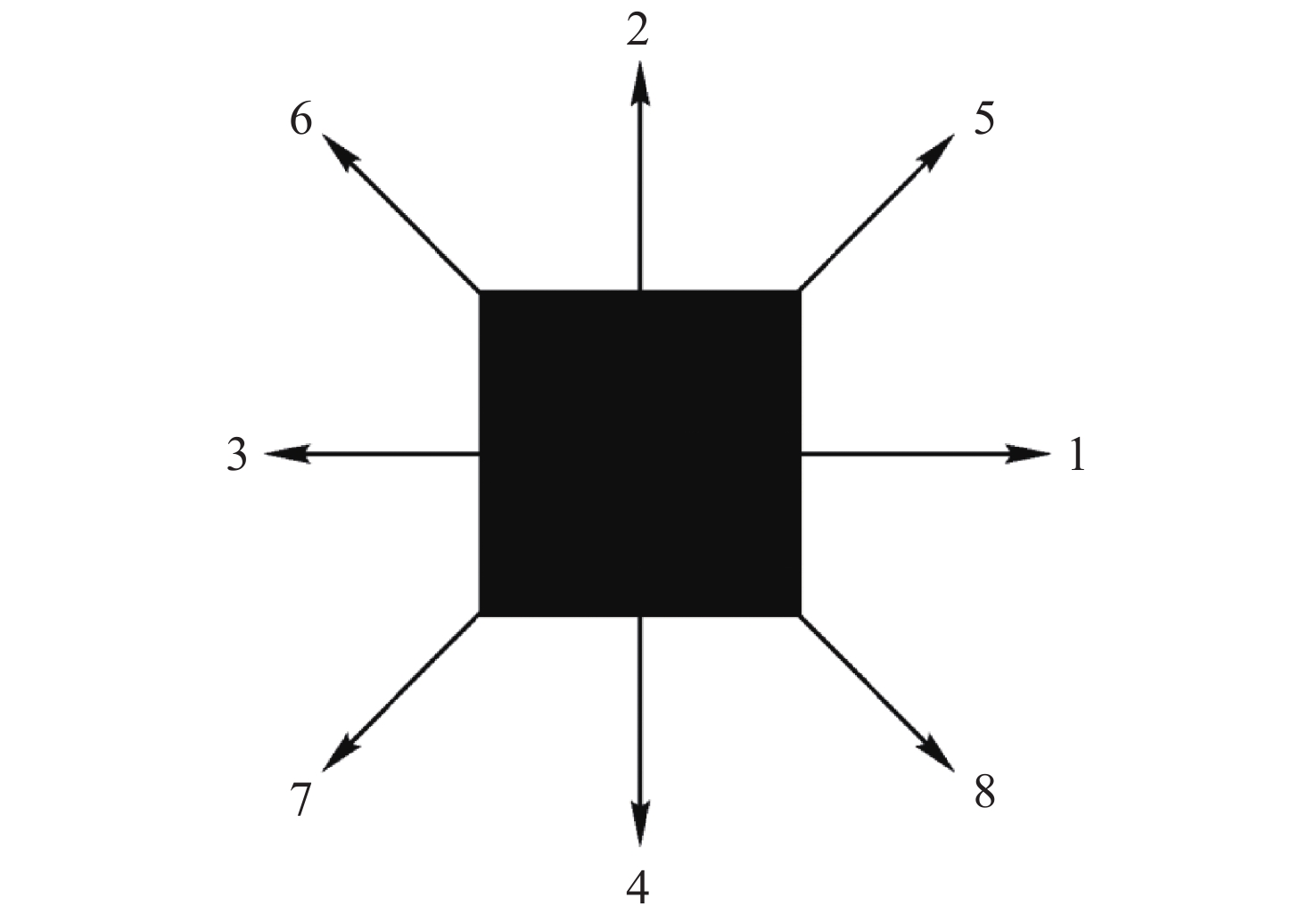
Figure 1.
Eight growth directions of each cell for randomly-generated 2-D porous media
-
Figure 2.
Diagram of a geometric model for simulation
-
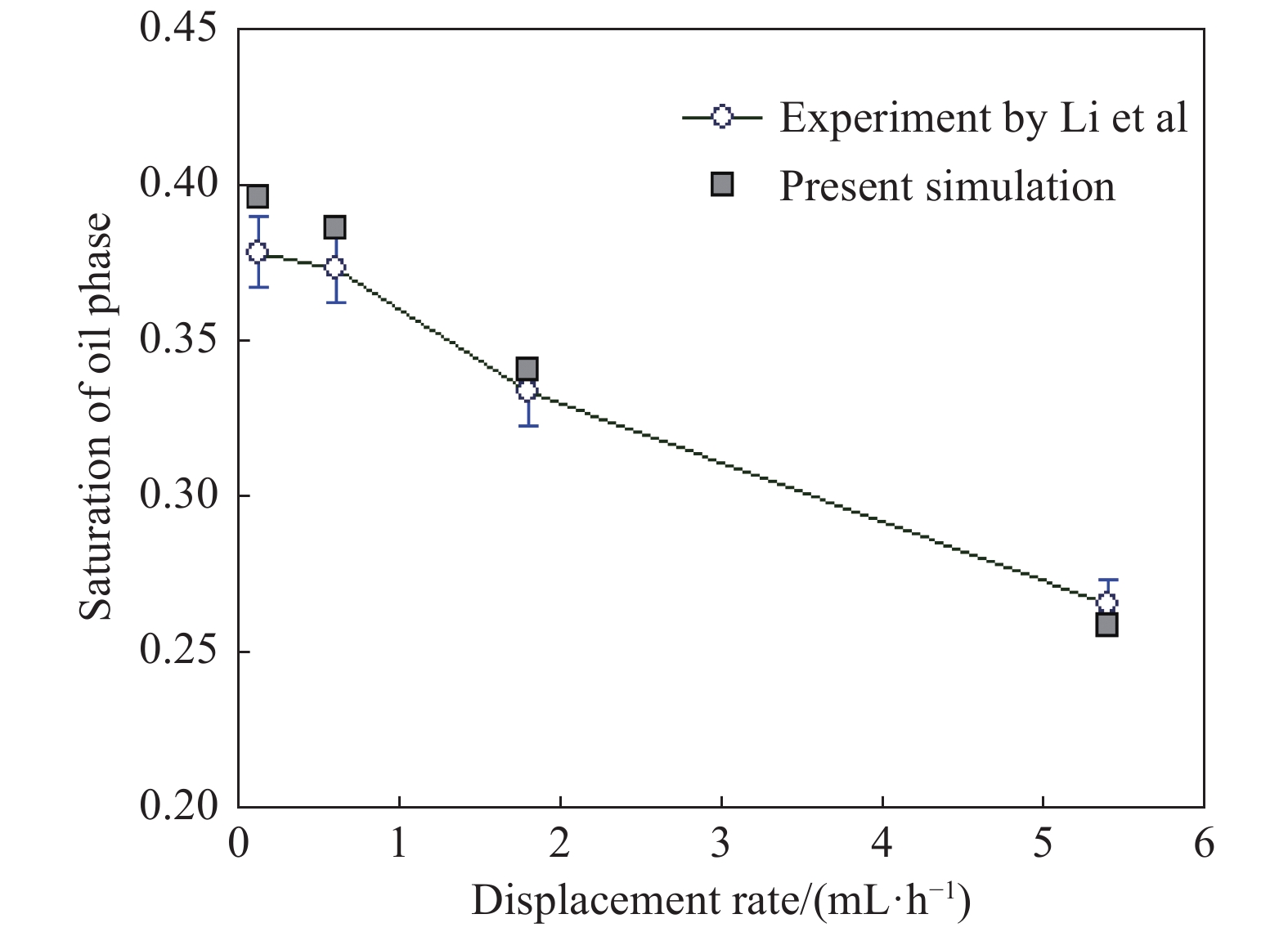
Figure 3.
Comparison of the outlet oil phase saturation between the simulation and experimental results
-
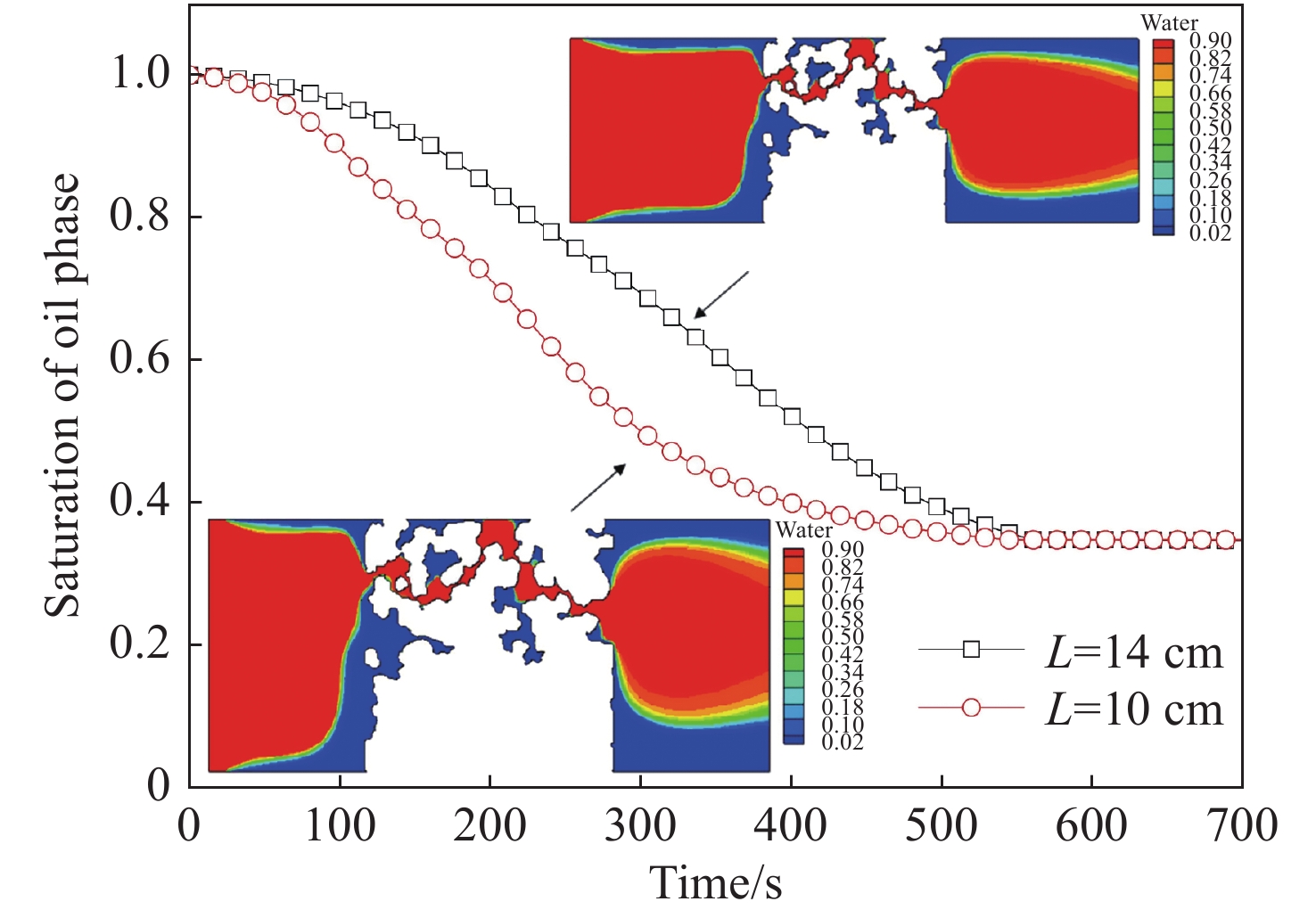
Figure 4.
Comparison of simulation results under different sizes of computational domain
-
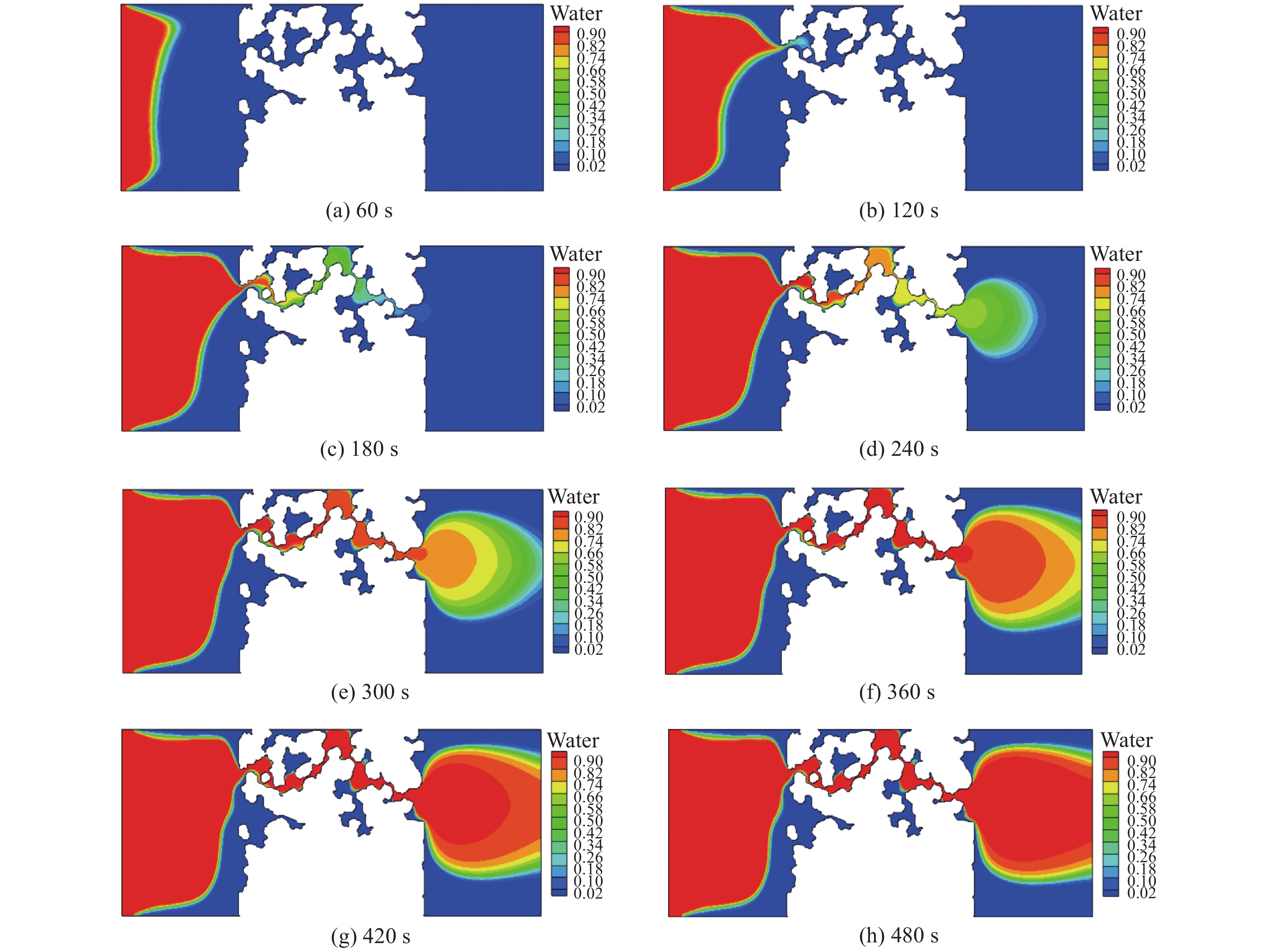
Figure 5.
Distribution of water phase during the process of oil displacement (Re=14.8)
-
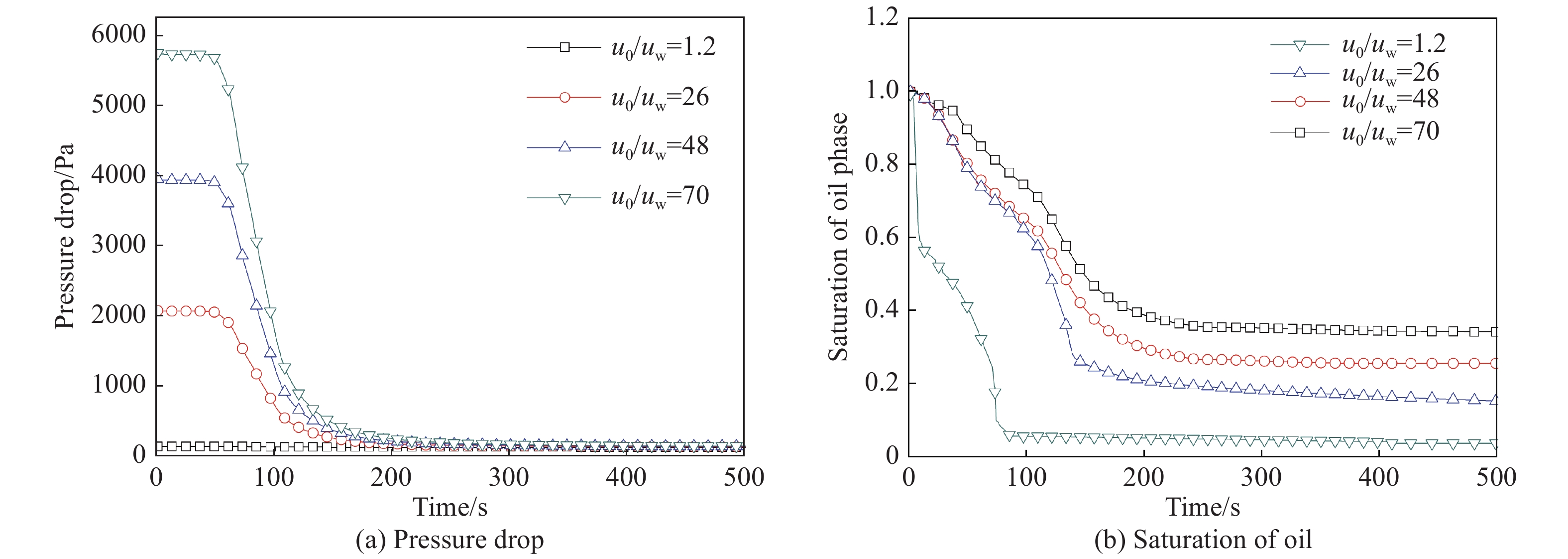
Figure 6.
Variations of the pressure drop and the saturation of oil phase between the inlet and outlet under different displacement velocities of water phase
-
Figure 7.
Variations of the pressure drop and the saturation of oil phase between the inlet and outlet under different viscosity ratios of oil-water phase
-
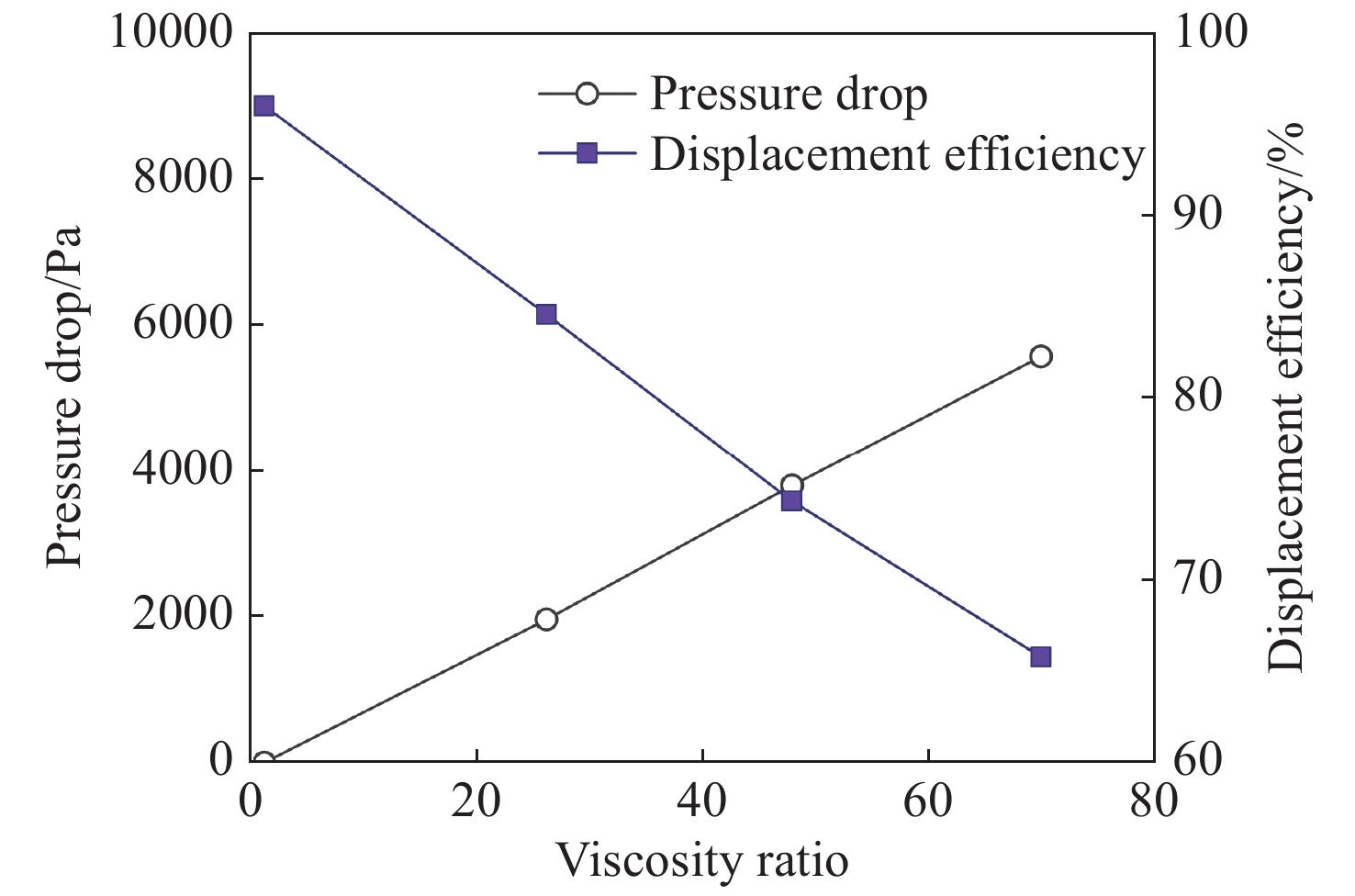
Figure 8.
Variations of the pressure drop and displacement efficiency with oil-water viscosity ratios
-
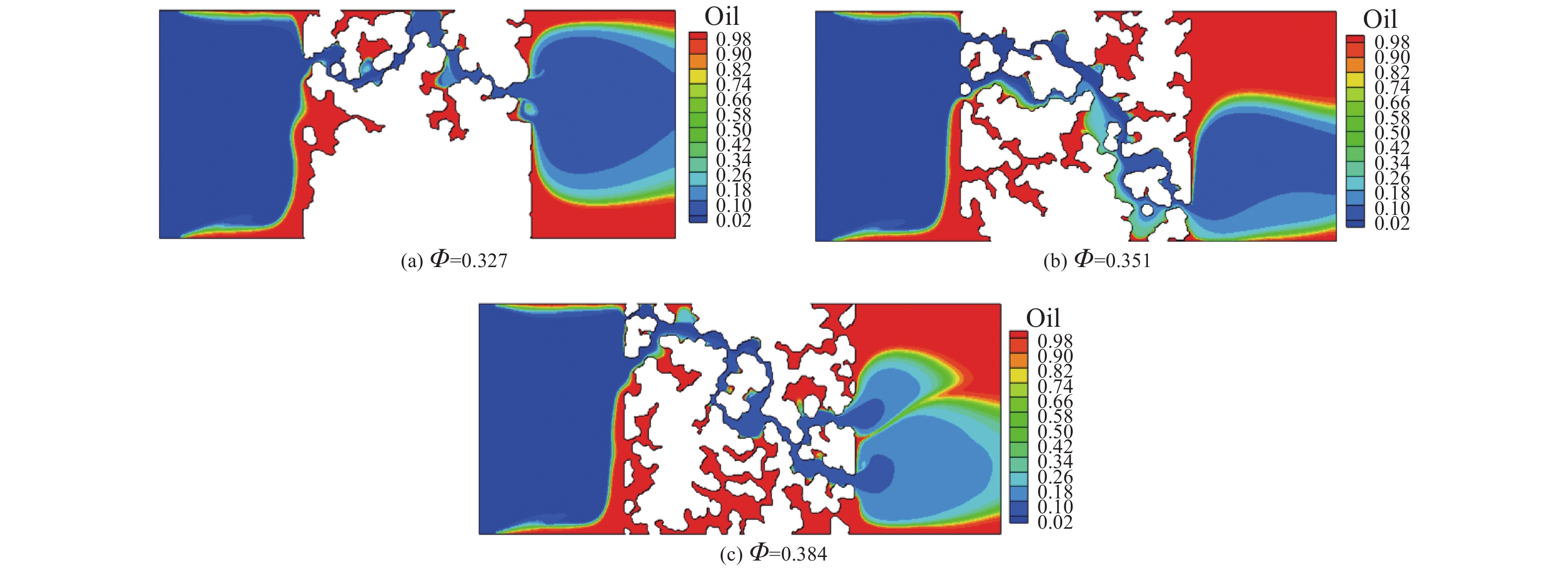
Figure 9.
Distribution of oil phase at 180 s during the process of oil displacement (Re=58.7)
-
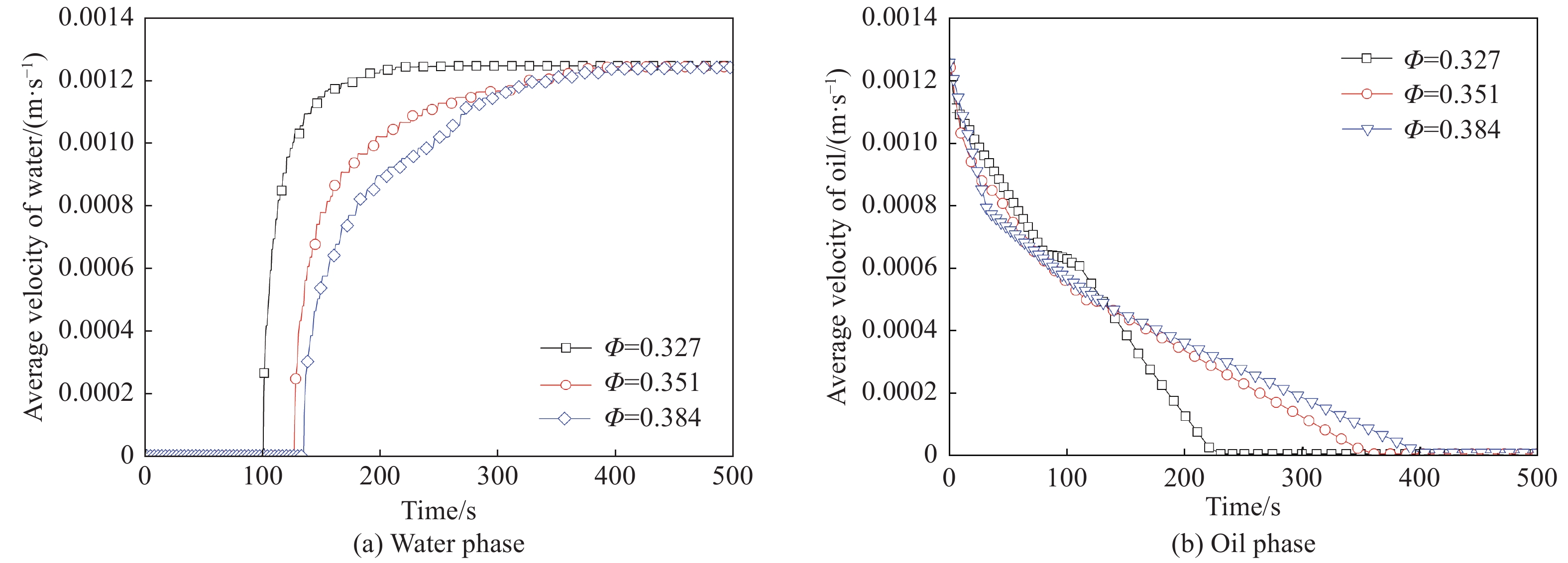
Figure 10.
Variations of the average velocity of water and oil phase under different porosities
-
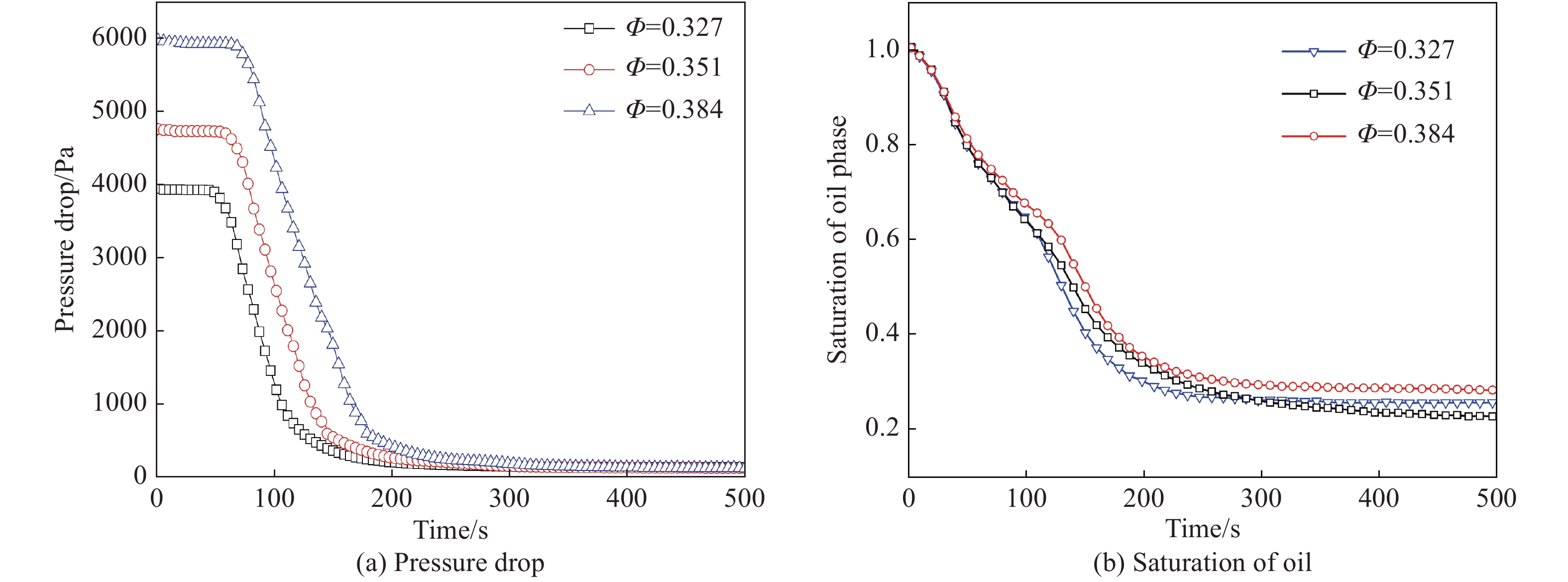
Figure 11.
Variations of the pressure drop and the saturation of oil phase between the inlet and outlet under different porosities
-
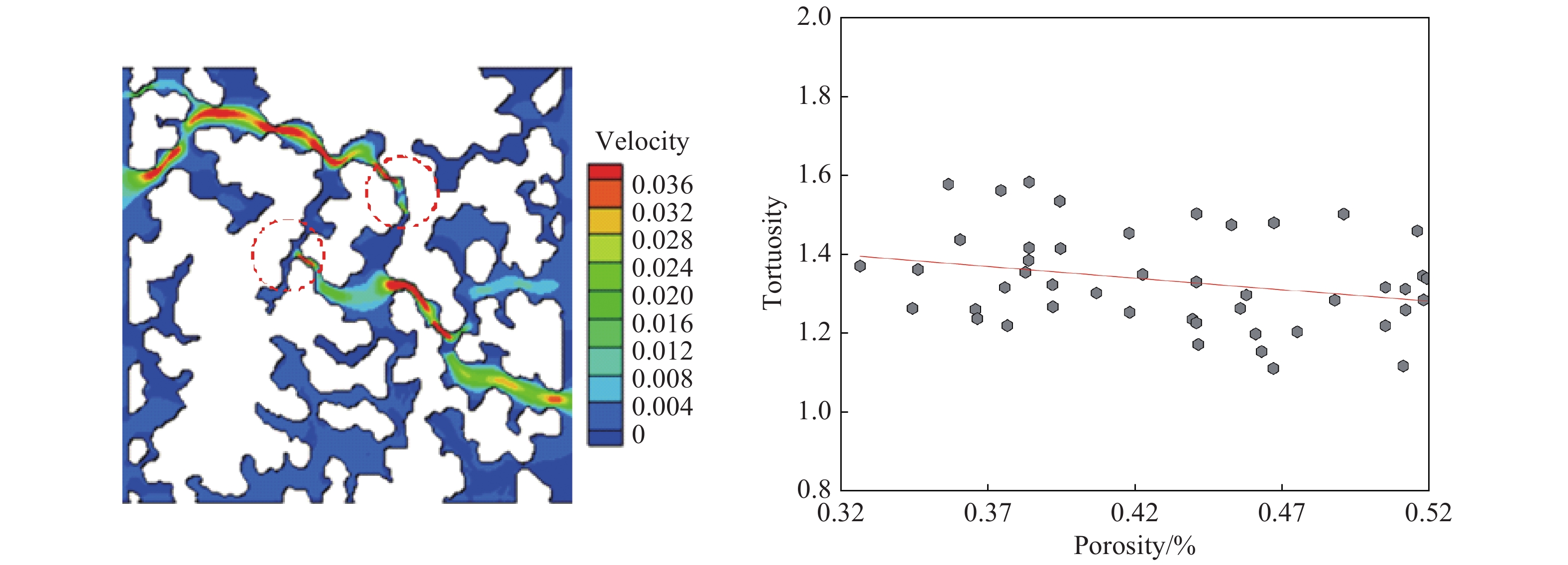
Figure 12.
Fine throats along preferential flow paths (Φ=0.384) and variations of tortuosity with porosity of porous media with random pores